Detector of thunderstorm danger

The third part of the article "Lightning protection of aircrafts"

Calculated data similar to those presented in fig. 5, can be received for each certain aircraft, considering its shape and sizes. And if it is so, why not to try and create an on-board device for control of the electric field along the flight course? There are no serious problems with the measuring. Modern gauss meters (the detectors of electric field) are able to measure the electric intensity from units of volts per meter. The problem is not in measuring but in the representation of the results obtained. It's time to study the simplest situation. Let the aircraft fly strictly to the center of a distant thunder cell. Its charge Q is unknown, like the distance from the aircraft R. We will use the results of two measures of the electric field E₁ and E₂, which were made with the time interval, during which the measuring system sensor approached the charged center to the distance Δr. At the known value of Δr it is enough to define the charge of a thunder cell and its distance to the aircraft. In fact, joint solution of equations wrote according to Coulomb law

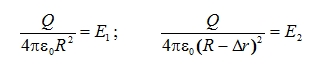
formula-2

(2)

gives

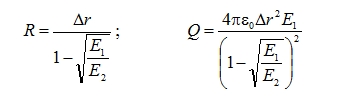
formula-3

(3)

Both these values are crucial. Charge value allows to estimate the speed of the electric field growth on drawing near it, and the current distance to the charge is necessary for a timely turn sart, if there is a danger and it is necessary to avoid the thunder center. For modern large-scale aircrafts with high response time, the warning should be made a dozen of kilometers before. For an arbitrary orientation of an aircraft regarding the charged center, at least 3 field sensors registrating laterla, transverse and vertical components of E vector are required. The volume of calculations will increase, but there wont be any special difficulties. It's a pity that a single charged center is an absolutely unreal situation for a thunder undercast. Even in the simplest environment, a cloud presents itself a dipole charge. Closer to the ground with the center on the height of 3-4 jm, a negatively charged area is located, and the positive is located 2-3 km higher. In case of approximately equal charges, a charged dipole with the Q charge and arm d=2-3 km is formed (fig. 6).

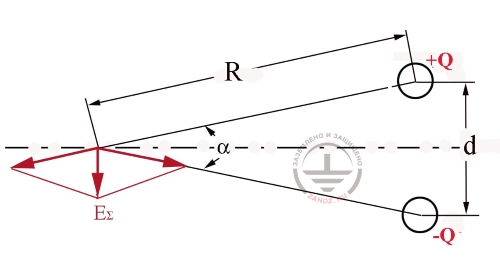
fig. 6. The field of the charged dipole at a great distance from its charges

Elementary structures in fig. 6 show the estimation of its field 6 EΣ at a great distance R. Now EΣ vector is directed perpendicular to the direction of the aicraft flight, and its value changes in reverse proportion to the cube of the distance to the charged centers:

Registration of a quickly declining field requires high sensibility of sensors. Besides, at the expense of parameter d, the number of the unknown and consequently, the number of measures, which should be processed for their determination. It is left to add some cloud charge to this image in order to make the task more complex. Let it be, for example, negative and be located directly above the aircraft, completely corrupting not only the value but the direction of vector EΣ. The task becomes insoluble, because in reality the number of charged centers in the undercast is now known to anybody. That is why, there are still no fully-featured on-board means of warning about thunder danger, and the control of situation by clutters on on-board sonar is not always effective. It is impossible to avoid straight impact of lightning.

E. M. Bazelyan, DEA, professor
Energy Institute named after G.M. Krzyzanowski, Moscow

See also: